
Kelly, for instance, reads 8 pages of a book every day.
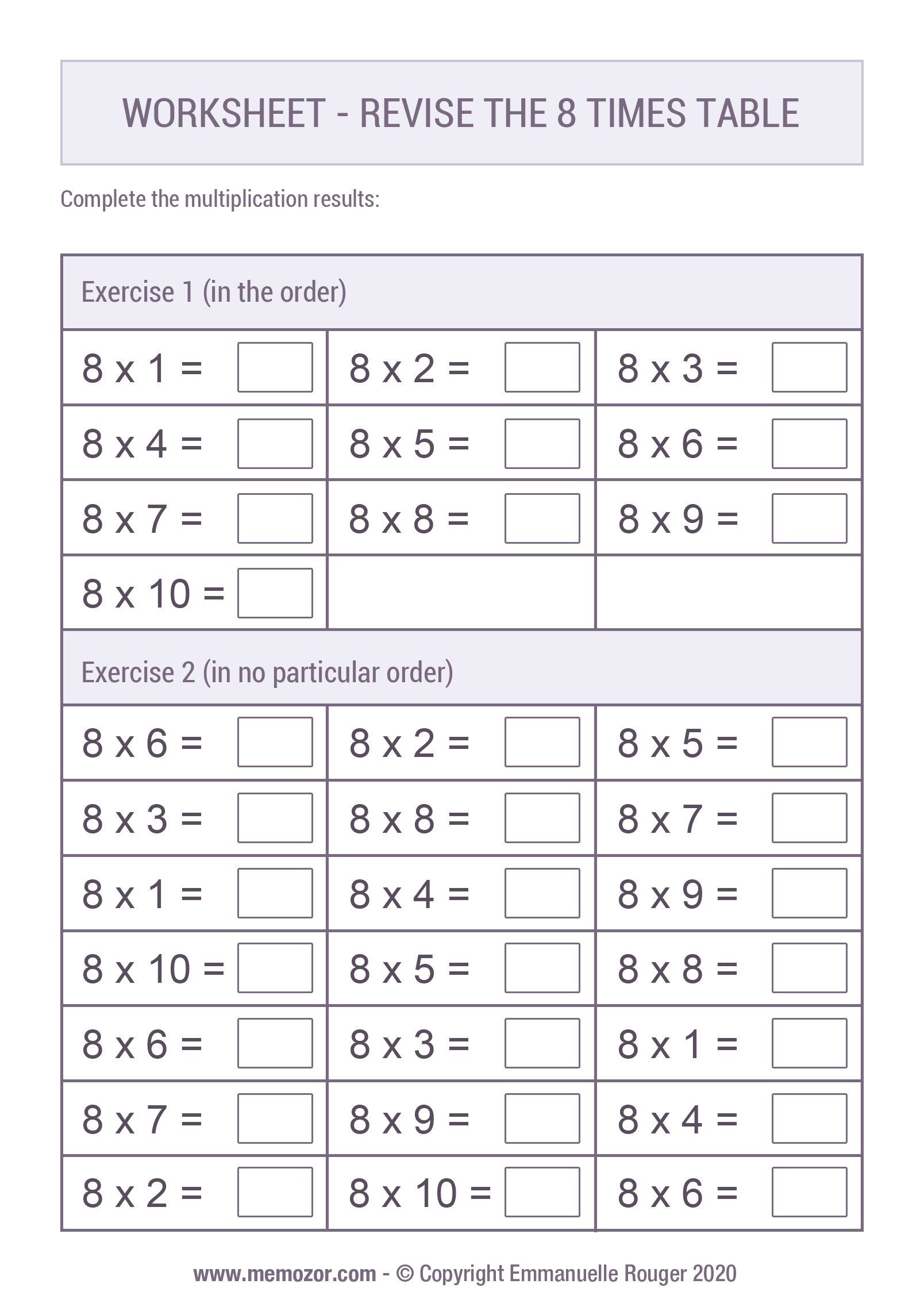
#8 times table how to#
You will learn how to memorize an eight-times table in this lesson, which is an eight-by-eight multiplication table containing whole numbers up to twenty. An intriguing property of the number 8 is that, when turned over, it represents infinity (). Students who can understand the table of eights are more confident in their mathematical skills. Memory is a transferable talent that will help students throughout their educational careers and lives, and learning the 8 times table will help them get better at it. So this list is the list of prime numbers between 10 and 20.The results of multiplying 8 by various whole numbers are displayed in the 8 table. In the case of 11, 13, 17, and 19, this means that they do not factor at all, except as a product of 1 and themselves. So a number not in the table can not be factored as a product of two single digit numbers. The multiplication table contains all products of single digit numbers. We see that 10, 12, 14, 15, 16, 18, and 20 all appear in the table so the missing numbers between 10 and 20 are The number 72 will eventually appear 12 times and the number 48 will appear 10 times. If the table were continued further, other larger numbers such as 72 and 48 would appear many more than 4 times. The numbers which appear 4 times have two distinct factorizations into single digit numbers (up to the order of the factors): for example, $24 = 4 \times 6 = 3 \times 8$. There are some numbers, such as 9, 16, and 36, which appear 3 times. Several numbers appear 4 times in the table: 6, 8, 12, 18, and 24.

If we extended the table to include products of all numbers, then every number $n$ (including all perfect squares) would appear at least twice as $n\times1$ and $1\times n$. Some products on the diagonal, such as 2 $\times$ 2 = 4, do also appear elsewhere because they have a different factorization (4 $\times$ 1 and 1 $\times$ 4 in this case). This is the commutative property of multiplication and several of these squares are shaded below:įor a number on the diagonal like $49 = 7 \times 7$, the two factors are the same and so when we switch their order, we don't get a different entry in the table. This makes sense because a number such as 48 = 6 $\times$ 8, not on the diagonal, also appears as 48 = 8 $\times$ 6. These squares are each shaded in different colors in the picture below: These are all along the diagonal of the table. The numbers which only appear once in the table are 1, 25, 49, 64, and 81. MP3, Construct Viable Arguments and Critique the Reasoning of Others. If students work on this task in groups and share their insights, then it is also a good opportunity to engage in MP7, Look For and Make Use of Structure, since the goal of the task is to help students understand the multiplication table from the point of view of factorizations. The main standard for mathematical practice which aligns with this task is The first non-prime number not appearing in the table is $22 = 2 \times 11$, the product of the smallest prime number with the smallest prime number bigger than 9.
This makes sense in the context because a prime number cannot be written as a product of two smaller whole numbers. The last part of this question gives an opportunity for the teacher to discuss prime numbers since the list of numbers the students produce will all be primes. Working through the table to see where different numbers appear, the students will have a good opportunity to observe the symmetry of the table which comes from the commutative property of multiplication: $a \times b = b \times a$. For example, 24 appears 4 times in the table:Ģ4 = 3 \times 8 = 8 \times 3 = 6 \times 4 = 4 \times 6.įor the 9 by 9 multiplication table shown, only the numbers 1 through 9 appear with all of their factorizations. The table shows some, but not necessarily all, factorizations of different numbers. The goal of this task is to encourage students to study the multiplication table, a familiar object, from a novel point of view.


 0 kommentar(er)
0 kommentar(er)
